This is a long post, it helps you understand options a bit better and make some profitable decisions in the future! I try to make theta and options easier to understand by relating it to real estate and moving one step at a time.
Let’s dive into the world of Theta and options trading!!
#1: Theta is a feature of an option. Plain and simple
magine showing someone a house and saying to them “It’s 3 bedrooms, 2 washrooms, good neighborhood, and the rent is $2,000/mo”.
Each thing you listed in that sentence is a feature of the house. You wouldn’t say that the house is good or bad because bedroom, or because rent, etc. It’s the over all view with all things considered.
Similarly, theta, gamma, vega, delta, etc… these are just features of an option. Inherently, they are not good or bad. they just tell you about the option you are looking at.
#2: Think of theta as rent
If options were a house, theta would be the rent. Think about it like this. Someone pays rent to get access to the house.
In the options space: someone pays theta for access to other features of the option.
Can you guess what they are paying for access to?
#3: One man’s theta is another man’s gamma
If you guessed gamma, you are correct! Traders pay theta to get access to gamma.
The easy way to think about gamma is that it’s your sensitivity to big moves.
If a stock moves like crazy, the option buyer makes some bank, right? So why on earth would ANYONE sell options?
#4: The amount of theta is directly correlated with the “gamma risk”
Going back to our house example, if you wanted to buy a big penthouse in downtown New York, the rent is probably pretty high. It’s because you get access to some awesome shit if you pay it! It wouldn’t make sense for the rent to be $500/mo. No one would rent it out! The rent is correlated with the house you get exposure to.
In the options space, if a stock has a lot of “gamma risk”, AKA the risk of big move, the theta on the option is higher too! This is because if it were not proportionately higher, no one would be a seller, and there would be no market.
Now heres the key. If gamma and theta were perfectly even, and markets were totally efficient, the expected value would be 0 (you wouldn’t make money being a buyer or seller). In this world, who wouldn’t want break even exposure to big moves?? It’s basically a free hedge!
SO…. there’s this little thing called variance risk premium
#5 Option sellers get a small premium for being on the short side of convexity
The variance risk premium is a small edge for the option seller that they get for holding the risk of big moves.
Because of this, on average, selling options is profitable. In the long run, you will have a lot of small winners and the occasional big loser. This is what we call a “short vol” strategy.
You can see the risk premium on a lot of stocks. An easy way to see it is to plot the Implied volatility for 30 day options over the realized volatility for 30 day period. You should see that on average the implied move (what the options SAY will happen), is a bit higher than what actually does. THIS IS THE PREMIUM!
And then you will see periods where the big gamma move happens, and the RV goes higher than the IV. THIS IS WHY THE PREMIUM EXISTS!
Here is an example:

Green Line = IV. Blue line = RV. this is on SPY. You can see how most of the time, IV > RV, Sometimes the RV Shoots up though. That is the risk we take when selling (why we get paid a premium)
But here’s the thing.. how much can we really expect to make here? In the long run, about 11% per year. I want more. You want more. Fuck 11% / year. So how do we do it?
#6 Buy Cheap Things, Sell Expensive Things
Let’s go back to our house example, 1 more time. Imagine we are evaluating a property in New York City. All of a sudden, a HUGE amount of demand comes into the market. There is a shortage of houses for all the renters, so the rent keeps increasing. You look at your property.. 2 beds, nice view.. fair rent is probably $4,000 /mo. But you look at the market and people are offering $6,000/mo for your property!!
In this case, by renting out your property, you are making an Inflated premium, or a rent premium higher than what you should be making given the asset you are giving someone access to.
In options, we can find stocks where the Theta is HIGHER than it should be, given the gamma we give someone exposure to! Think about some of these meme stocks as example. So many buyers, so few sellers (who wants that risk, right?).
Well… this is perhaps opportunity!
If we can come into the market and put a fair value on the “gamma”, we can find times where we can be overcompensated with theta.
There is a simple formula for understanding this:
IF IMPLIED VOLATILITY IS HIGHER THAN WHAT YOU THINK REALIZED VOLATILITY WILL BE: SELL!
Even more simply put: if option more expensive than how much the stock moves, sell! The hard part is learning to price volatility / options (I’ll cover the basics in another post if this one does well).
#7 Here’s an example of how I analyze/price gamma and theta
Imagine we divided the IV by the RV, we would be able to see how much higher or lower the IV is compared to the RV.
Consider this example: if IV/RV = 1.5, then the price of the option is 1.5x higher than the value the buyer is actually getting (easy way to think about it). By plotting the IV/RV Ratio historically, we can see how much of a premium their typically is, and how bad it gets when the gamma move is big!
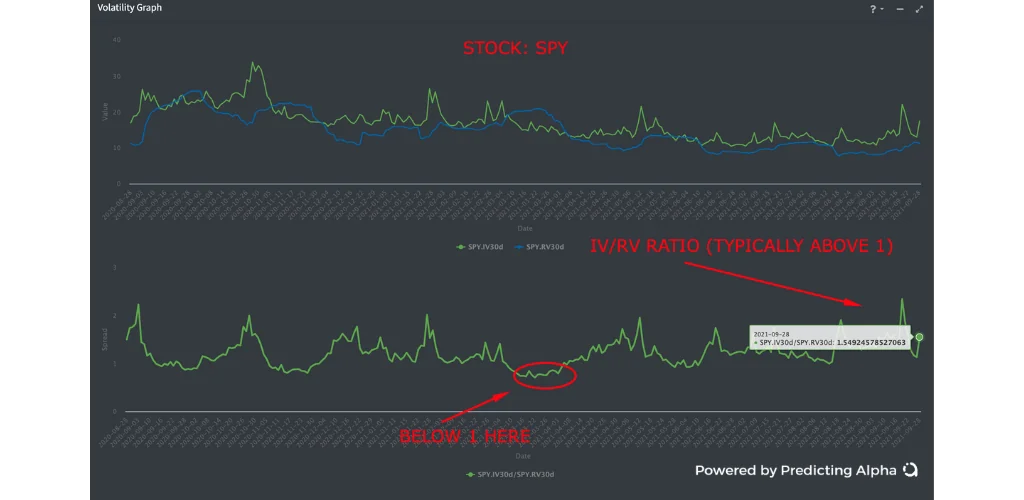
Typically the iv/rv ratio for 30 DTE options is positive on SPY but we do have periods of pain where it goes lower and that erases a lot of gains.
Expected Value of Trade Decision Making
This section will show you how profitable traders think about the trades and strategies they are looking at.
In the other part of this guide, we discussed how to think about the value of the options we are trading. We learned about risk premium, selling when things are expensive, and how to actually determine if something IS expensive.
In this part, we are going to tie this all together by introducing the mentality that profitable traders have when thinking about trading.
A challenge with trading
One of the characteristics of trading that makes it challenging is that in every strategy (profitable ones and unprofitable ones) there is a lot of variance.
Over the long run, it’s not reasonable for us to expect our PnL graphs to be a beautiful straight line from the bottom left of the screen all the way to the top right. It’d be nice… but it’s not going to happen unfortunately.
Instead, our PnL is filled with periods of making money, losing money, and not much happening at all.
This trading characteristic comes with a pro and a con.
- Pro: Losing money today doesn’t mean you have a bad strategy
- Con: Making money today doesn’t mean you have a good strategy.
Take this chart for example:
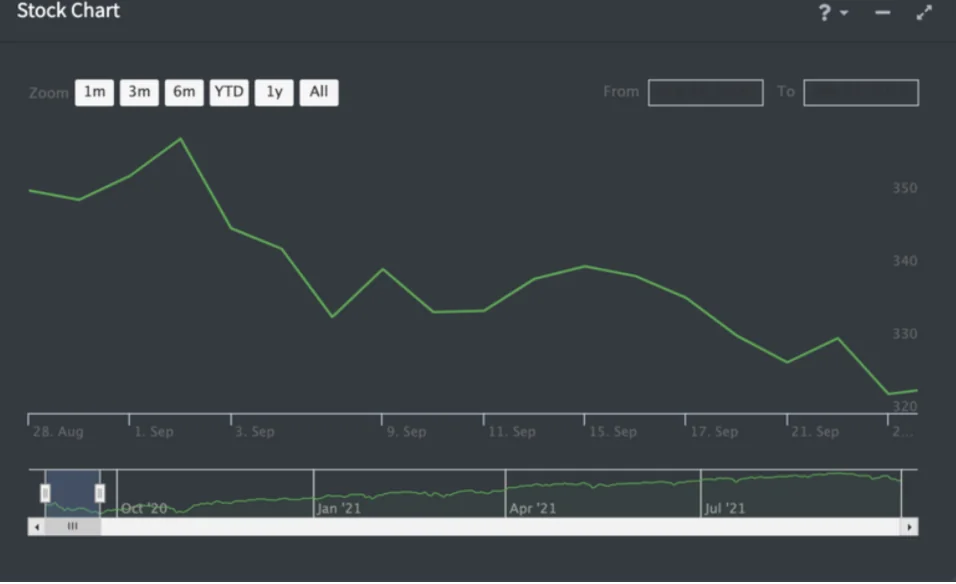
This is a price chart for a stock. Imagine this was your entire portfolio holdings.
If this were your strategy, it looks awful, right? In just one month there is almost a 10% drawdown! But if we zoom out and look at a long time frame.

Thinking strategically about strategy
“Not every good trade is profitable. Not every profitable trade is good.”
Even when you put in a lot of work and have the utmost confidence in an idea, you can still lose money on it. But that doesn’t mean it was a bad idea, or that you shouldn’t take similar trades in the future.
If your analysis was as good as you believe it to be, taking those trades over and over again into the future could be extremely lucrative, even though there are some losers.
In order to see beyond our one trade, we need to be thinking in terms of the expected value of our strategy.
What is expected value?
Expected value is a betting concept that helps us answer this question:
“If I were to take this bet/trade over and over again into the future, how much money will I make or lose?”
It is how professional traders look at their trades because it allows them to quickly and easily assess whether the trade they are looking at is worth taking.
There is actually a formula for calculating the expected value of a strategy. This is what it is.
Your expected value of a bet is equal to the probability of you winning multiplied by how much you make if you win. Then you subtract the probability of you losing multiplied by how much you are risking on the bet.
Here is the Expected Value formula:

We always want to be taking trades that have a positive expected value. A positive expected value tells us that a trade, on average, has a profitable return. And if we keep taking that bet again and again into the future, the average return per trade (total return divided by total bets placed) should be our expected value.
How does having a positive expected value impact our trading?
Think of it like having a weighted coin, where there is a 51% chance of landing on heads. If you only flip the coin once, it is basically 50/50. But the more you flip the coin, the more you will start to see a bias towards heads. To the point where if you flip the coin thousands of times there will be a huge bias towards the number of times the coin lands on heads.
Example 1: Casino expected value
Here is an example of what expected value looks like if you owned a casino.
Let’s set a scenario. Imagine you are the dealer at a blackjack table. Every time someone plays, they bet $10. If the house wins, they take the $10, and if the gambler wins, they make $10 profit. In blackjack, the casino has a 1% edge over the gambler, or a 51% chance of winning. So what’s the expected value? Let’s plug the numbers in and find out.

As you can see, the casino’s expected value per bet, is $0.20 cents.
But if any of you have spent time at a casino you know the following: On any given bet, winning or losing is pretty much 50/50. But in the long run, it seems like everyone is down money… except for the casino.
This is the expected value at work. And to help realize their expected value, the casino controls the size of the bet (doesn’t let you bet big enough to take them out), and they have many games going on at the same time to increase the frequency of betting.
Let’s do another example to see how expected value plays out in the real world.
Example 2: The Coin Toss
In this example we are going to do a simulation of people tossing a weighted coin. This means that the coin has a 51% chance of landing on heads, 49% chance of lading on tail. The participants will bet $10 per coin toss, and the R:R is 1:1. ( risk 10 to make 10).
Let’s see what happens. I simulated this data using the r statistical programming language.
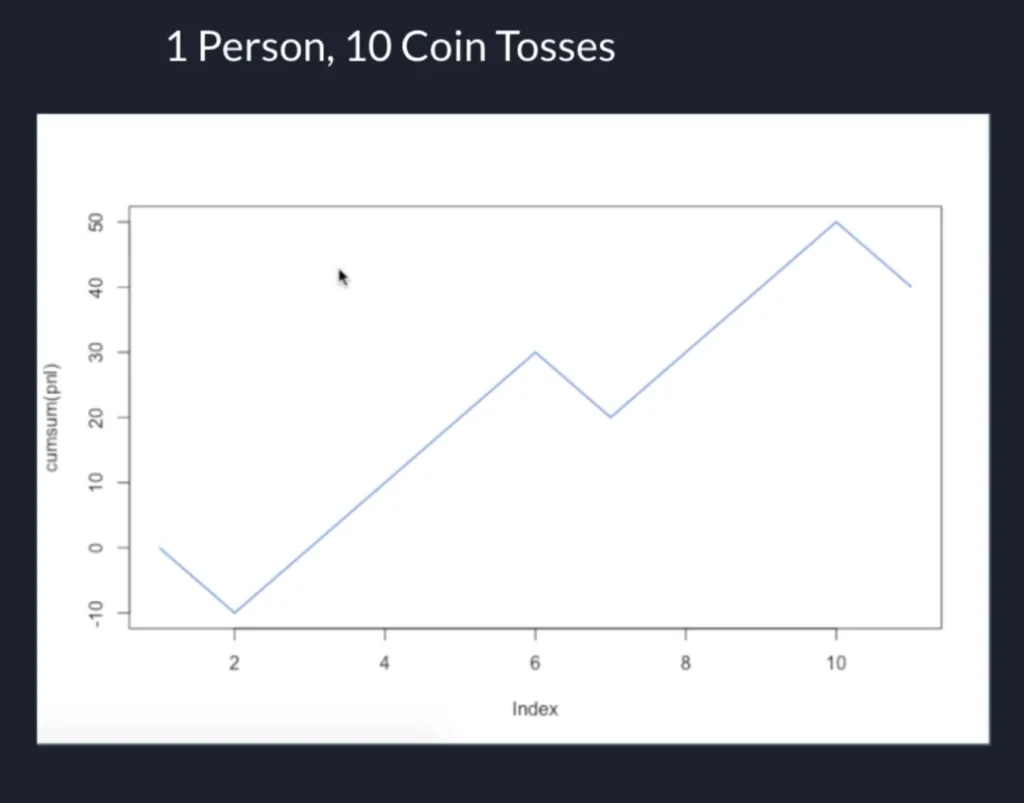
The first person tosses the coin 10 times. Check this out! He made some money. So do we run this strategy now? Is this proof of our expected value?
Maybe not quite yet.. Because check out what happens when 10 people toss the coin 10 times.
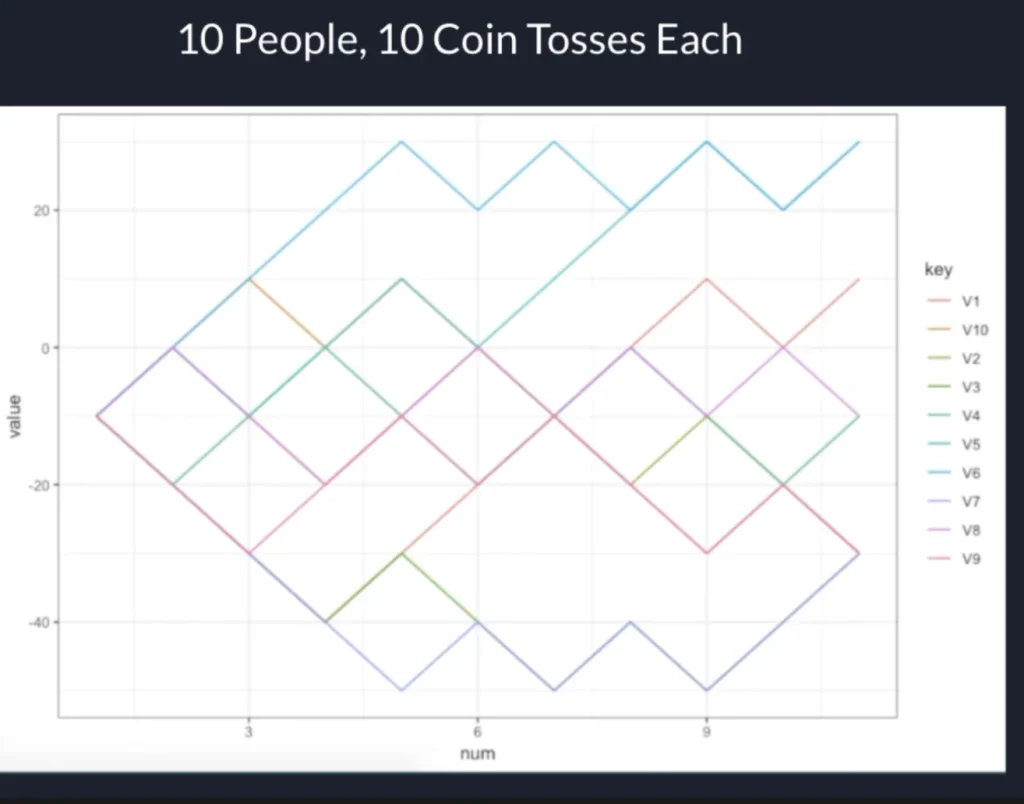
Some people make money.. Some people lose money. It looks pretty random. Maybe the expected value is a load of BS! To find out.. Let’s increase the number of times these 10 people toss the coin.
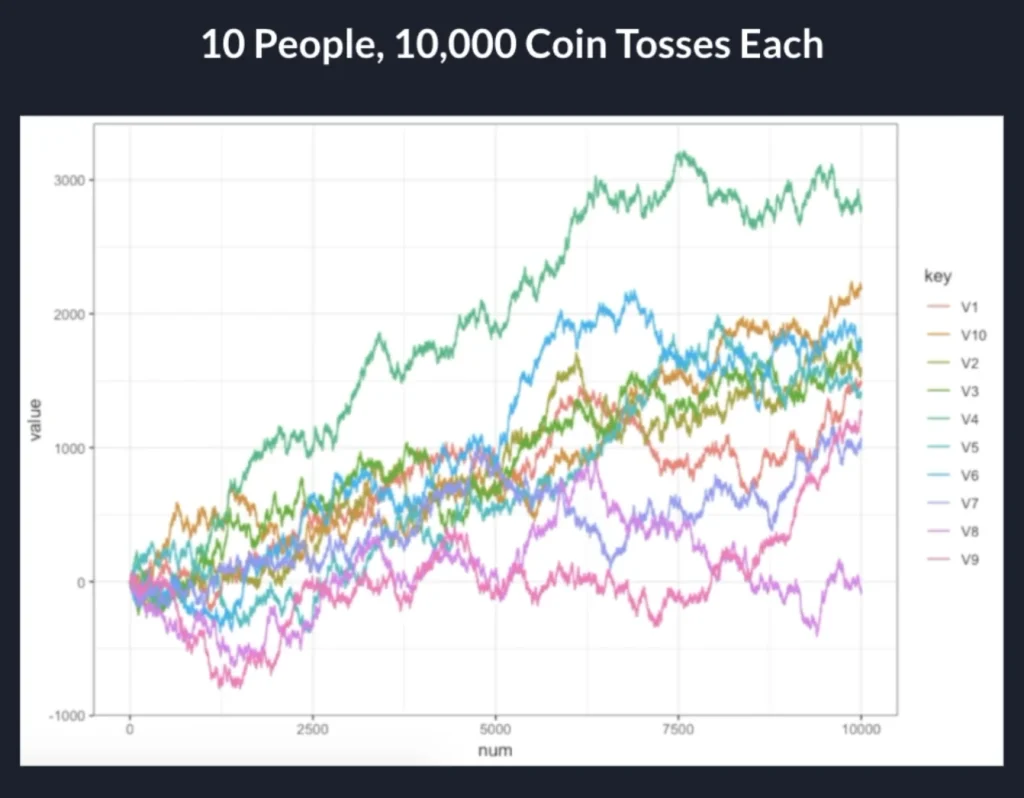
WOW! At 10,000 coin tosses, 9/10 people a pretty crazy return! One person lost a bit of money. Let’s see what happens if we up it to 100,000 tosses:
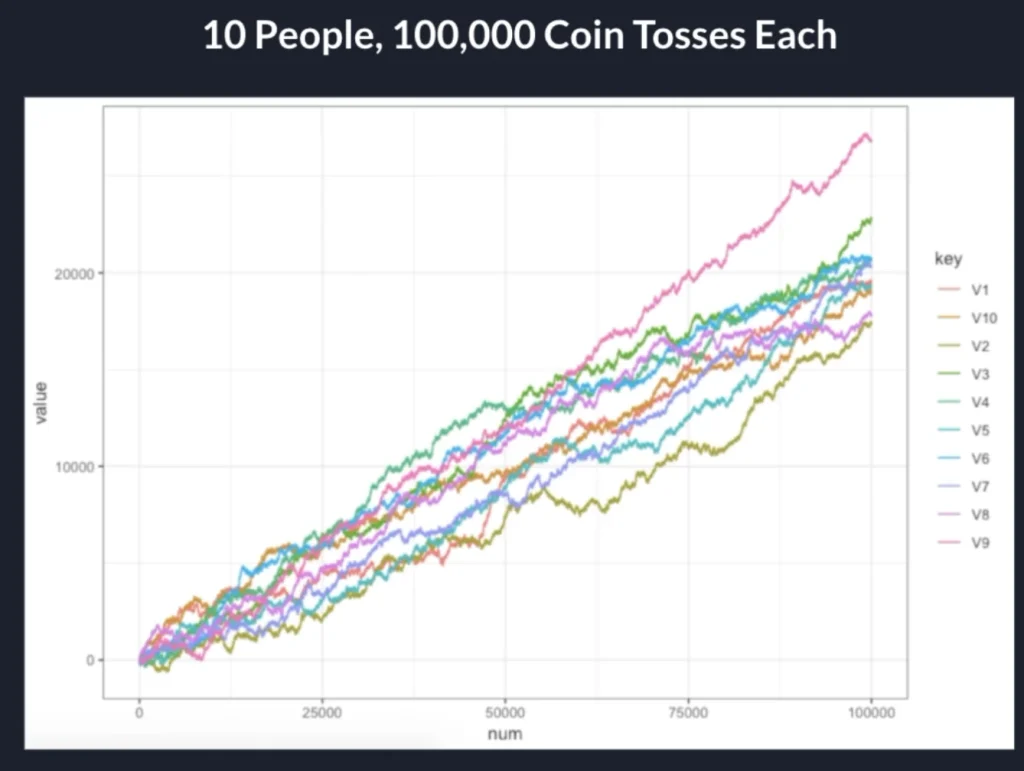
My god, at 100,000 tosses EVERYONE who played this game got rich.
So as you can see.. A small edge. JUST ONE PERCENT! Was enough to get rich.We don’t need to hit home runs every day to make money as traders. We just need a little advantage that gives us that positive expected value.
Now obviously, knowing that you have an edge and being able to really quantify it is trickier than this post makes it seem. But what’s important right now is to at least start thinking this way.
Tying expected value back to some basic options trading strategies
When we look at the market, what do you think the expected value of a trade should be if we are blindly trading? If the market were perfectly efficient, the EV should be 0!
The market isn’t on your team or my team. It doesn’t inherently try to give anyone an advantage. If the market were perfectly efficient, you shouldn’t really be making money buying OR selling!
Why is this important?
Remember this: The expected value of your strategy takes into consideration the probability of winning/losing and the risk reward of the trade.
So.. when we look at a random trade that has a high “probability of profit”.. What should that tell us about the risk ? if we have a 90% chance of winning, and the market tries to give a 0 EV bet.. That means our risk needs to be much greater than our reward!
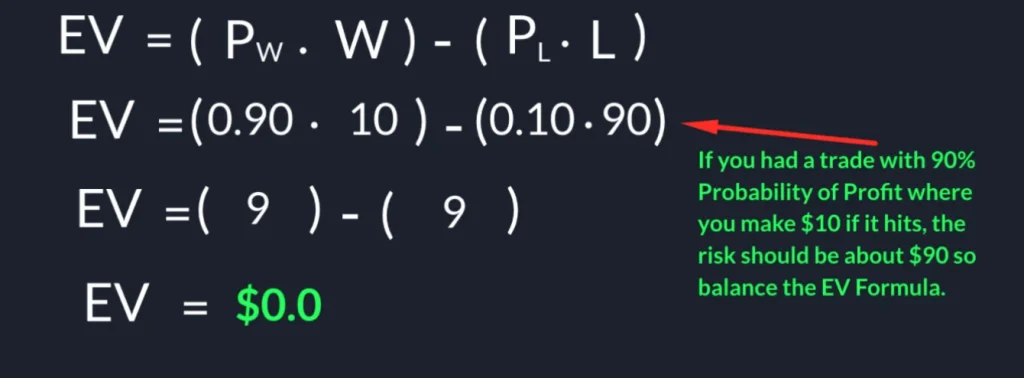
The same goes for strategies with low probabilities of profit, if you have a low chance of winning, the payoff should be bigger than the risk to make the EV 0. Keep this in mind when looking for trades. So with this understanding, think about this:
Is selling 0dte delta 20 strangles on SPY really free/easy money? Who is buying those things? What happens if the stock actually does move big?
According to the EV formula… The risk should really outweigh the reward here. Leading to sayings like “picking up pennies in front of a steamroller”.
Is the EV really 0 as a baseline in options?
The short answer is no.
The reason is because options are convex products (If you buy an option and the stock moves like crazy, the payoff is huge), and sellers take on very large exposure. If the EV were 0 , no one would want to sell, and the option buyer could get a free hedge on their portfolio. SO! There is a slightly positive EV for the seller to incentivize them to be there.
How much is the EV for option sellers?
On average, it is 11% / year! This is the Variance Risk Premium, which is the premium paid to option sellers for providing access to big move payoffs.
How else can we increase our expected value?
There’s a couple ways, but the one that has the biggest impact is finding an edge (topic for next post?). By being more thorough with our analysis, and really digging deep to get an advantage over other market participants, we can increase our returns significantly.
Conclusion
By having the expected value on your side, you are expected to win overall. And this is how we should look at trading. Any individual trade has a big luck component, but as long as we have an advantage in every trade we place, we end up making huge returns as we place more and more trades.
This is so important to understand because in the short term, it can be very hard to know if you are supposed to keep winning if you don’t know your expected value. It is extremely dangerous to play without thinking about the expected value, because if you don’t have it on your side, nothing else matters.
You could be amazing at managing risk, but you will still bleed out slowly over time. That’s what keeps losing traders coming back, because you don’t lose every time. You just so happen to lose more than you win.
But when you do have it on your side, you are setting yourself up for a lifetime of success. A casino wins games, loses games, but they don’t stress. The blackjack deal could be drunk for all we know, and it doesn’t matter. They still make their dollars and know exactly how that system’s expected value works.
If we can do that in our trading, we can build profitable strategies and find profitable trade ideas.
Happy Trading